MEAM_LAMMPS_KimJeonLee_2015_MgY__MO_018428823000_002
MEAM_LAMMPS_KimJeonLee_2015_MgY__MO_018428823000_002
| Title
A single sentence description.
|
MEAM Potential for the Mg-Y system developed by Kim, Jeon, and Lee (2015) v002 |
|---|---|
| Description
A short description of the Model describing its key features including for example: type of model (pair potential, 3-body potential, EAM, etc.), modeled species (Ac, Ag, ..., Zr), intended purpose, origin, and so on.
|
Interatomic potential Mg–Y binary system has been developed on the basis of the second nearest-neighbor modified embedded-atom method (2NN MEAM) formalism. The potential can describe the alloy behavior (structural, thermodynamic and defect properties of solid solutions and compounds) of binary system in reasonable agreement with experimental data or first-principles and other calculations. |
| Species
The supported atomic species.
| Mg, Y |
| Disclaimer
A statement of applicability provided by the contributor, informing users of the intended use of this KIM Item.
|
None |
| Content Origin | http://cmse.postech.ac.kr/home_2nnmeam |
| Contributor |
Jong-Kwan Lee |
| Maintainer |
Jong-Kwan Lee |
| Developer |
Ki-Hyun Kim Jong Bae Jeon Byeong-Joo Lee |
| Published on KIM | 2023 |
| How to Cite |
This Model originally published in [1] is archived in OpenKIM [2-5]. [1] Kim K-H, Jeon JB, Lee B-J. Modified embedded-atom method interatomic potentials for Mg–X (X= Y, Sn, Ca) binary systems. Calphad. 2015;48:27–34. doi:10.1016/j.calphad.2014.10.001 — (Primary Source) A primary source is a reference directly related to the item documenting its development, as opposed to other sources that are provided as background information. [2] Kim K-H, Jeon JB, Lee B-J. MEAM Potential for the Mg-Y system developed by Kim, Jeon, and Lee (2015) v002. OpenKIM; 2023. doi:10.25950/2bbc6ac9 [3] Afshar Y, Hütter S, Rudd RE, Stukowski A, Tipton WW, Trinkle DR, et al. The modified embedded atom method (MEAM) potential v002. OpenKIM; 2023. doi:10.25950/ee5eba52 [4] Tadmor EB, Elliott RS, Sethna JP, Miller RE, Becker CA. The potential of atomistic simulations and the Knowledgebase of Interatomic Models. JOM. 2011;63(7):17. doi:10.1007/s11837-011-0102-6 [5] Elliott RS, Tadmor EB. Knowledgebase of Interatomic Models (KIM) Application Programming Interface (API). OpenKIM; 2011. doi:10.25950/ff8f563a Click here to download the above citation in BibTeX format. |
| Citations
This panel presents information regarding the papers that have cited the interatomic potential (IP) whose page you are on. The OpenKIM machine learning based Deep Citation framework is used to determine whether the citing article actually used the IP in computations (denoted by "USED") or only provides it as a background citation (denoted by "NOT USED"). For more details on Deep Citation and how to work with this panel, click the documentation link at the top of the panel. The word cloud to the right is generated from the abstracts of IP principle source(s) (given below in "How to Cite") and the citing articles that were determined to have used the IP in order to provide users with a quick sense of the types of physical phenomena to which this IP is applied. The bar chart shows the number of articles that cited the IP per year. Each bar is divided into green (articles that USED the IP) and blue (articles that did NOT USE the IP). Users are encouraged to correct Deep Citation errors in determination by clicking the speech icon next to a citing article and providing updated information. This will be integrated into the next Deep Citation learning cycle, which occurs on a regular basis. OpenKIM acknowledges the support of the Allen Institute for AI through the Semantic Scholar project for providing citation information and full text of articles when available, which are used to train the Deep Citation ML algorithm. |
This panel provides information on past usage of this interatomic potential (IP) powered by the OpenKIM Deep Citation framework. The word cloud indicates typical applications of the potential. The bar chart shows citations per year of this IP (bars are divided into articles that used the IP (green) and those that did not (blue)). The complete list of articles that cited this IP is provided below along with the Deep Citation determination on usage. See the Deep Citation documentation for more information. 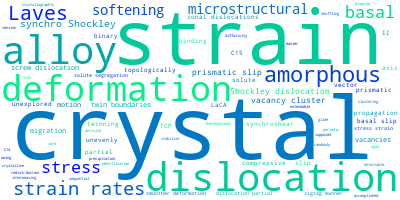
68 Citations (57 used)
Help us to determine which of the papers that cite this potential actually used it to perform calculations. If you know, click the .
USED (high confidence) Z. Xie, D. Chauraud, A. Atila, E. Bitzek, S. Korte-Kerzel, and J. Gu’enol’e, “Thermally activated nature of synchro-Shockley dislocations in Laves phases,” Scripta Materialia. 2023. link Times cited: 3 USED (high confidence) Y. Hu and D. Kochmann, “Atomistic insight into three-dimensional twin embryo growth in Mg alloys,” Journal of Materials Science. 2023. link Times cited: 1 USED (high confidence) Z. Xie, D. Chauraud, A. Atila, E. Bitzek, S. Korte-Kerzel, and J. Gu’enol’e, “Unveiling the mechanisms of motion of synchro-Shockley dislocations in Laves phases,” Physical Review Materials. 2022. link Times cited: 5 Abstract: In Laves phases, synchroshear is the dominant basal slip mec… read more USED (high confidence) P. Yi, T. Sasaki, S. Prameela, T. Weihs, and M. Falk, “The Interplay between Solute Atoms and Vacancy Clusters in Magnesium Alloys,” SSRN Electronic Journal. 2021. link Times cited: 2 Abstract: Atomic-scale calculations indicate that both stress effects … read more USED (high confidence) Z. Xie, D. Chauraud, E. Bitzek, S. Korte-Kerzel, and J. Gu’enol’e, “Laves phase crystal analysis (LaCA): Atomistic identification of lattice defects in C14 and C15 topologically close-packed phases,” Journal of Materials Research. 2021. link Times cited: 6 Abstract: The identification of defects in crystal structures is cruci… read more USED (high confidence) J.-K. Lee and B.-J. Lee, “The Origin of Activation of Non-basal Slip in Mg-Ce Dilute Alloy: An Atomistic Simulation Study,” Metallurgical and Materials Transactions A. 2021. link Times cited: 9 USED (high confidence) Z. Huang et al., “Dislocation-induced Y segregation at basal-prismatic interfaces in Mg,” arXiv: Materials Science. 2020. link Times cited: 7 USED (high confidence) Y. Dou, H. Luo, and J. Zhang, “The effects of yttrium on the 10-12 twinning behaviour in magnesium alloys: a molecular dynamics study,” Philosophical Magazine Letters. 2020. link Times cited: 3 Abstract: ABSTRACT {10-12} twinning is one of the most important defor… read more USED (high confidence) S. Wang, H. Pan, P. Wang, and F.-guo Zhang, “Microstructural evolution of single-crystal magnesium under elevated temperature and ultra-high strain rate,” Journal of Applied Physics. 2019. link Times cited: 6 Abstract: Despite numerous studies of the deformation behavior of magn… read more USED (high confidence) J. Gu’enol’e, F. Mouhib, L. Huber, B. Grabowski, and S. Korte-Kerzel, “Basal slip in Laves phases: The synchroshear dislocation,” Scripta Materialia. 2019. link Times cited: 34 USED (high confidence) Peng 鹏 Yi 易, R. Cammarata, and M. Falk, “Solute softening and defect generation during prismatic slip in magnesium alloys,” Modelling and Simulation in Materials Science and Engineering. 2017. link Times cited: 16 Abstract: Temperature and solute effects on prismatic slip of 〈a〉 disl… read more USED (high confidence) H. Fan, J.-J. Tang, X. Tian, Q. Wang, X. Tian, and J. El-Awady, “Core structures and mobility of ⟨c⟩ dislocations in magnesium,” Scripta Materialia. 2017. link Times cited: 21 USED (high confidence) G. Zu and S. Groh, “Effect of segregated alloying element on the intrinsic fracture behavior of Mg,” Theoretical and Applied Fracture Mechanics. 2016. link Times cited: 3 USED (high confidence) H. Fan and J. El-Awady, “Towards resolving the anonymity of Pyramidal slip in magnesium,” Materials Science and Engineering A-structural Materials Properties Microstructure and Processing. 2015. link Times cited: 66 USED (low confidence) D. G. Kizzire et al., “Modified embedded atom method interatomic potential for FCC γ-cerium,” Computational Materials Science. 2023. link Times cited: 0 USED (low confidence) L. Liu, M. Miyake, L. Li, W. Jia, and Y. Shibutani, “Numerical study on the ductility improvement mechanism of dilute Mg-Ca alloys,” Computational Materials Science. 2023. link Times cited: 1 USED (low confidence) L. Liu, M. Miyake, T. Matsuda, L. Li, and Y. Shibutani, “Analytical model for estimating calcium solution strengthening in single-crystal Mg-Ca alloys at finite temperatures,” Materialia. 2023. link Times cited: 0 USED (low confidence) L. Han, H. Y. Song, M. An, T. Shen, and Y. Li, “A novel strengthening mechanism in crystalline/amorphous dual-phase Mg alloys: A molecular dynamics study,” Journal of Non-Crystalline Solids. 2023. link Times cited: 2 USED (low confidence) S. Li, H. Y. Song, L. Han, and W. Su, “Effect of rare earth element yttrium on migration behavior of twin boundary in magnesium alloys: A molecular dynamics study,” Journal of Materials Research and Technology. 2023. link Times cited: 3 USED (low confidence) F. Gao, Q. Yang, J. Du, and G. Jiang, “Mechanical Behavior and Physical Properties of Mg Binary Alloys via Y-doping: Molecular Dynamic Study,” Journal of Materials Engineering and Performance. 2023. link Times cited: 0 USED (low confidence) G. Shen et al., “Effect of nano-CaO particle on the microstructure, mechanical properties and corrosion behavior of lean Mg-1Zn alloy,” Journal of Magnesium and Alloys. 2023. link Times cited: 4 USED (low confidence) S. Gowthaman and T. Jagadeesha, “Impact of Point Defects on the Creep Behavior of MgY Polycrystal:A Molecular Dynamics Study,” Materials Letters. 2022. link Times cited: 1 USED (low confidence) J. Du, H. Y. Song, M. An, and Y. Li, “Effect of rare earth element on amorphization and deformation behavior of crystalline/amorphous dual-phase Mg alloys,” Materials & Design. 2022. link Times cited: 5 USED (low confidence) L. Han et al., “Neural network potential for studying the thermal conductivity of Sn,” Computational Materials Science. 2021. link Times cited: 8 USED (low confidence) A. Maldar et al., “Activation of dislocations in Mg with solute Y,” Journal of Magnesium and Alloys. 2021. link Times cited: 10 USED (low confidence) J.-J. Tang et al., “Interaction between dislocation loop and 101¯2 twin boundary in magnesium,” Journal of Nuclear Materials. 2021. link Times cited: 4 USED (low confidence) X. Zhou, H. Su, H. Ye, and Z. Yang, “Removing basal-dissociated 〈c+a〉 dislocations by 101¯2 deformation twinning in magnesium alloys,” Acta Materialia. 2021. link Times cited: 17 USED (low confidence) Z. Li, X. Tian, J.-J. Tang, Q. Wang, W. Jiang, and H. Fan, “Molecular dynamics simulations on the dislocation interactions in magnesium,” Computational Materials Science. 2021. link Times cited: 2 USED (low confidence) H.-S. Jang, J.-K. Lee, A. J. Tapia, N. Kim, and B.-J. Lee, “Activation of non-basal slip in multicomponent Mg alloys,” Journal of Magnesium and Alloys. 2021. link Times cited: 23 USED (low confidence) X. Wu, C. Li, C. Guo, and Z. Du, “Thermodynamic re-assessment of the Mg–Y binary system coupling with the precipitation sequence during aging process,” Calphad-computer Coupling of Phase Diagrams and Thermochemistry. 2020. link Times cited: 7 USED (low confidence) J. Gu’enol’e, M. Zubair, S. Roy, Z. Xie, S. Sandlobes-Haut, and S. Korte-Kerzel, “Exploring the transfer of plasticity across Laves phase interfaces in a dual phase magnesium alloy,” Materials & Design. 2020. link Times cited: 14 USED (low confidence) Y. Zhang et al., “Shuffle and glide mechanisms of prismatic dislocations in a hexagonal
C14
-type Laves-phase intermetallic compound,” Physical Review B. 2020. link Times cited: 8 USED (low confidence) H.-S. Jang, D. Seol, and B.-J. Lee, “Modified embedded-atom method interatomic potentials for Mg–Al–Ca and Mg–Al–Zn ternary systems,” Journal of Magnesium and Alloys. 2020. link Times cited: 28 USED (low confidence) R. Boom and F. D. Boer, “Enthalpy of formation of binary solid and liquid Mg alloys – Comparison of Miedema-model calculations with data reported in literature,” Calphad-computer Coupling of Phase Diagrams and Thermochemistry. 2020. link Times cited: 4 USED (low confidence) R. Ahmad, Z. Wu, and W. Curtin, “Analysis of double cross-slip of pyramidal I 〈c+a〉 screw dislocations and implications for ductility in Mg alloys,” Acta Materialia. 2020. link Times cited: 65 USED (low confidence) S. Wang, H. Pan, A. He, P. Wang, and F.-guo Zhang, “Amorphous structure in single-crystal magnesium under compression along the
c
axis with ultrahigh strain rate,” Physical Review B. 2019. link Times cited: 3 USED (low confidence) H.-S. Jang, D. Seol, and B.-J. Lee, “Modified embedded-atom method interatomic potential for the Mg–Zn–Ca ternary system,” Calphad-computer Coupling of Phase Diagrams and Thermochemistry. 2019. link Times cited: 6 USED (low confidence) M. Zacate, “Modified embedded-atom method potential for cadmium,” Hyperfine Interactions. 2019. link Times cited: 0 USED (low confidence) P. Yi and M. Falk, “Thermally activated twin thickening and solute softening in magnesium alloys - a molecular simulation study,” Scripta Materialia. 2019. link Times cited: 11 USED (low confidence) R. Mohammadzadeh and M. Mohammadzadeh, “Effect of Ca addition on plastic flow in nanocrystalline magnesium by atomistic simulation,” Computational Materials Science. 2019. link Times cited: 6 USED (low confidence) H. Fan, Y. Zhu, and Q. Wang, “Effect of precipitate orientation on the twinning deformation in magnesium alloys,” Computational Materials Science. 2018. link Times cited: 19 USED (low confidence) Z. Pei, “An overview of modeling the stacking faults in lightweight and high-entropy alloys: Theory and application,” Materials Science and Engineering: A. 2018. link Times cited: 33 USED (low confidence) S. Ju and C.-C. Yang, “Understanding the structural, mechanical, thermal, and electronic properties of MgCa bulk metallic glasses by molecular dynamics simulation and density functional theory calculation,” Computational Materials Science. 2018. link Times cited: 5 USED (low confidence) R. Ahmad, Z. Wu, S. Groh, and W. Curtin, “Pyramidal II to basal transformation of ⟨ + ⟩ edge dislocations in Mg-Y alloys,” Scripta Materialia. 2018. link Times cited: 24 USED (low confidence) Z. Pei, H. Sheng, X. Zhang, R. Li, and B. Svendsen, “Tunable twin stability and an accurate magnesium interatomic potential for dislocation-twin interactions,” Materials & Design. 2018. link Times cited: 17 USED (low confidence) H. Fan, Y. Zhu, J. El-Awady, and D. Raabe, “Precipitation hardening effects on extension twinning in magnesium alloys,” International Journal of Plasticity. 2018. link Times cited: 86 USED (low confidence) H.-S. Jang, K.-M. Kim, and B.-J. Lee, “Modified embedded-atom method interatomic potentials for pure Zn and Mg-Zn binary system,” Calphad-computer Coupling of Phase Diagrams and Thermochemistry. 2018. link Times cited: 23 USED (low confidence) C. Barrett, A. Imandoust, and H. Kadiri, “The effect of rare earth element segregation on grain boundary energy and mobility in magnesium and ensuing texture weakening,” Scripta Materialia. 2018. link Times cited: 94 USED (low confidence) K. Kim, J.-H. Hwang, H.-S. Jang, J. Jeon, N. Kim, and B.-J. Lee, “Dislocation binding as an origin for the improvement of room temperature ductility in Mg alloys,” Materials Science and Engineering A-structural Materials Properties Microstructure and Processing. 2018. link Times cited: 54 USED (low confidence) K. Kim and B.-J. Lee, “Modified embedded-atom method interatomic potentials for Mg-Nd and Mg-Pb binary systems,” Calphad-computer Coupling of Phase Diagrams and Thermochemistry. 2017. link Times cited: 12 USED (low confidence) H. Fan, Q. Wang, X. Tian, and J. El-Awady, “Temperature effects on the mobility of pyramidal dislocations in magnesium,” Scripta Materialia. 2017. link Times cited: 59 USED (low confidence) R. Reddy and S. Groh, “Atomistic modeling of the effect of calcium on the yield surface of nanopolycrystalline magnesium-based alloys,” Computational Materials Science. 2016. link Times cited: 10 USED (low confidence) K. Kim, J. Jeon, N. Kim, and B.-J. Lee, “Role of yttrium in activation of 〈c + a〉 slip in magnesium: An atomistic approach,” Scripta Materialia. 2015. link Times cited: 101 USED (low confidence) M. Alam and S. Groh, “Dislocation modeling in bcc lithium: A comparison between continuum and atomistic predictions in the modified embedded atoms method,” Journal of Physics and Chemistry of Solids. 2015. link Times cited: 19 USED (low confidence) Z. Xing, H. Fan, J.-J. Tang, B. Wang, and G. Kang, “Molecular dynamics simulation on the cyclic deformation of magnesium single crystals,” Computational Materials Science. 2021. link Times cited: 22 USED (low confidence) K. Srivastava and J. El-Awady, “The Effect of the Orientation of Second-Order Pyramidal Dislocations on Plastic Flow in Magnesium,” The Minerals, Metals & Materials Series. 2019. link Times cited: 1 USED (low confidence) S. Groh and M. K. Nahhas, “Modeling Dislocation in Binary Magnesium-Based Alloys Using Atomistic Method,” Handbook of Mechanics of Materials. 2019. link Times cited: 1 NOT USED (low confidence) A. Verma and S. Ogata, “Magnesium based alloys for reinforcing biopolymer composites and coatings: A critical overview on biomedical materials,” Advanced Industrial and Engineering Polymer Research. 2023. link Times cited: 6 NOT USED (low confidence) H.-S. Jang, K. Kim, N. Kim, and B.-J. Lee, “Understanding on the Role of Rare Earth Elements in Activation of $ \left⟨\textc + \texta \right⟩$ Slip in Magnesium: An Atomistic Approach.” 2017. link Times cited: 0 NOT USED (high confidence) T. Brink, L. Langenohl, H. Bishara, and G. Dehm, “Universality of grain boundary phases in fcc metals: Case study on high-angle [111] symmetric tilt grain boundaries,” Physical Review B. 2022. link Times cited: 6 Abstract: Grain boundaries often exhibit ordered atomic structures. In… read more NOT USED (high confidence) M. Çeltek, “Saf Kalsiyum Elementinin Isıtma Sürecinin Moleküler Dinamik Benzetim Yöntemi ile İncelenmesi,” Bitlis Eren Üniversitesi Fen Bilimleri Dergisi. 2021. link Times cited: 0 Abstract: In the study, the structural and some physical properties of… read more NOT USED (high confidence) S.-J. Sun, S. Ju, C.-C. Yang, K.-C. Chang, and I.-J. Lee, “Effects of Strontium incorporation to Mg-Zn-Ca biodegradable bulk metallic glass investigated by molecular dynamics simulation and density functional theory calculation,” Scientific Reports. 2020. link Times cited: 6 NOT USED (high confidence) J. Gu’enol’e et al., “Assessment and optimization of the fast inertial relaxation engine (fire) for energy minimization in atomistic simulations and its implementation in lammps,” Computational Materials Science. 2019. link Times cited: 89 NOT USED (high confidence) W. Ibarra-Hernández, S. Hajinazar, G. Avendaño-Franco, A. Bautista-Hernández, A. Kolmogorov, and A. Romero, “Structural search for stable Mg-Ca alloys accelerated with a neural network interatomic model.,” Physical chemistry chemical physics : PCCP. 2018. link Times cited: 14 Abstract: We have combined a neural network formalism with metaheurist… read more NOT USED (high confidence) R. Ahmad, S. Groh, M. Ghazisaeidi, and W. Curtin, “Modified embedded-atom method interatomic potential for Mg–Y alloys,” Modelling and Simulation in Materials Science and Engineering. 2018. link Times cited: 12 Abstract: An interatomic potential for the Mg–Y binary system is devel… read more NOT USED (high confidence) M. K. Nahhas and S. Groh, “Atomistic modeling of grain boundary behavior under shear conditions in magnesium and magnesium-based binary alloys,” Journal of Physics and Chemistry of Solids. 2018. link Times cited: 12 |
| Funding | Not available |
| Short KIM ID
The unique KIM identifier code.
| MO_018428823000_002 |
| Extended KIM ID
The long form of the KIM ID including a human readable prefix (100 characters max), two underscores, and the Short KIM ID. Extended KIM IDs can only contain alpha-numeric characters (letters and digits) and underscores and must begin with a letter.
| MEAM_LAMMPS_KimJeonLee_2015_MgY__MO_018428823000_002 |
| DOI |
10.25950/2bbc6ac9 https://doi.org/10.25950/2bbc6ac9 https://commons.datacite.org/doi.org/10.25950/2bbc6ac9 |
| KIM Item Type
Specifies whether this is a Portable Model (software implementation of an interatomic model); Portable Model with parameter file (parameter file to be read in by a Model Driver); Model Driver (software implementation of an interatomic model that reads in parameters).
| Portable Model using Model Driver MEAM_LAMMPS__MD_249792265679_002 |
| Driver | MEAM_LAMMPS__MD_249792265679_002 |
| KIM API Version | 2.2 |
| Potential Type | meam |
| Previous Version | MEAM_LAMMPS_KimJeonLee_2015_MgY__MO_018428823000_001 |
| Grade | Name | Category | Brief Description | Full Results | Aux File(s) |
|---|---|---|---|---|---|
| P | vc-species-supported-as-stated | mandatory | The model supports all species it claims to support; see full description. |
Results | Files |
| P | vc-periodicity-support | mandatory | Periodic boundary conditions are handled correctly; see full description. |
Results | Files |
| P | vc-permutation-symmetry | mandatory | Total energy and forces are unchanged when swapping atoms of the same species; see full description. |
Results | Files |
| A | vc-forces-numerical-derivative | consistency | Forces computed by the model agree with numerical derivatives of the energy; see full description. |
Results | Files |
| F | vc-dimer-continuity-c1 | informational | The energy versus separation relation of a pair of atoms is C1 continuous (i.e. the function and its first derivative are continuous); see full description. |
Results | Files |
| P | vc-objectivity | informational | Total energy is unchanged and forces transform correctly under rigid-body translation and rotation; see full description. |
Results | Files |
| P | vc-inversion-symmetry | informational | Total energy is unchanged and forces change sign when inverting a configuration through the origin; see full description. |
Results | Files |
| N/A | vc-memory-leak | informational | The model code does not have memory leaks (i.e. it releases all allocated memory at the end); see full description. |
Results | Files |
| P | vc-thread-safe | mandatory | The model returns the same energy and forces when computed in serial and when using parallel threads for a set of configurations. Note that this is not a guarantee of thread safety; see full description. |
Results | Files |
| P | vc-unit-conversion | mandatory | The model is able to correctly convert its energy and/or forces to different unit sets; see full description. |
Results | Files |
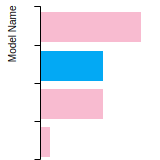
BCC Lattice Constant
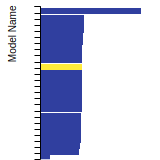
This bar chart plot shows the mono-atomic body-centered cubic (bcc) lattice constant predicted by the current model (shown in the unique color) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
Cohesive Energy Graph
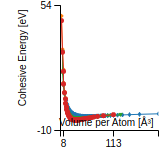
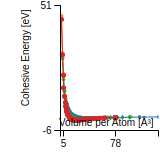
This graph shows the cohesive energy versus volume-per-atom for the current mode for four mono-atomic cubic phases (body-centered cubic (bcc), face-centered cubic (fcc), simple cubic (sc), and diamond). The curve with the lowest minimum is the ground state of the crystal if stable. (The crystal structure is enforced in these calculations, so the phase may not be stable.) Graphs are generated for each species supported by the model.
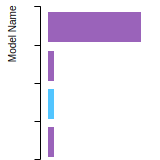
Diamond Lattice Constant
This bar chart plot shows the mono-atomic face-centered diamond lattice constant predicted by the current model (shown in the unique color) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
Dislocation Core Energies
This graph shows the dislocation core energy of a cubic crystal at zero temperature and pressure for a specific set of dislocation core cutoff radii. After obtaining the total energy of the system from conjugate gradient minimizations, non-singular, isotropic and anisotropic elasticity are applied to obtain the dislocation core energy for each of these supercells with different dipole distances. Graphs are generated for each species supported by the model.
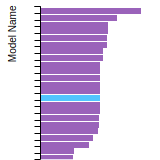
(No matching species)FCC Elastic Constants
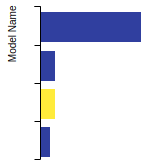
This bar chart plot shows the mono-atomic face-centered cubic (fcc) elastic constants predicted by the current model (shown in blue) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
FCC Lattice Constant
This bar chart plot shows the mono-atomic face-centered cubic (fcc) lattice constant predicted by the current model (shown in red) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
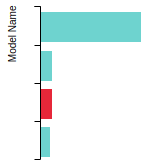
FCC Stacking Fault Energies
This bar chart plot shows the intrinsic and extrinsic stacking fault energies as well as the unstable stacking and unstable twinning energies for face-centered cubic (fcc) predicted by the current model (shown in blue) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
(No matching species)FCC Surface Energies
This bar chart plot shows the mono-atomic face-centered cubic (fcc) relaxed surface energies predicted by the current model (shown in blue) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
(No matching species)SC Lattice Constant
This bar chart plot shows the mono-atomic simple cubic (sc) lattice constant predicted by the current model (shown in the unique color) compared with the predictions for all other models in the OpenKIM Repository that support the species. The vertical bars show the average and standard deviation (one sigma) bounds for all model predictions. Graphs are generated for each species supported by the model.
Cubic Crystal Basic Properties Table
Species: MgSpecies: Y
Creators:
Contributor: karls
Publication Year: 2019
DOI: https://doi.org/10.25950/64cb38c5
This Test Driver uses LAMMPS to compute the cohesive energy of a given monoatomic cubic lattice (fcc, bcc, sc, or diamond) at a variety of lattice spacings. The lattice spacings range from a_min (=a_min_frac*a_0) to a_max (=a_max_frac*a_0) where a_0, a_min_frac, and a_max_frac are read from stdin (a_0 is typically approximately equal to the equilibrium lattice constant). The precise scaling and number of lattice spacings sampled between a_min and a_0 (a_0 and a_max) is specified by two additional parameters passed from stdin: N_lower and samplespacing_lower (N_upper and samplespacing_upper). Please see README.txt for further details.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Cohesive energy versus lattice constant curve for bcc Mg v004 | view | 8982 | |
| Cohesive energy versus lattice constant curve for bcc Y v004 | view | 7639 | |
| Cohesive energy versus lattice constant curve for diamond Mg v004 | view | 7957 | |
| Cohesive energy versus lattice constant curve for diamond Y v004 | view | 8524 | |
| Cohesive energy versus lattice constant curve for fcc Mg v004 | view | 8693 | |
| Cohesive energy versus lattice constant curve for fcc Y v004 | view | 8761 | |
| Cohesive energy versus lattice constant curve for sc Mg v004 | view | 8466 | |
| Cohesive energy versus lattice constant curve for sc Y v004 | view | 8614 |
Creators:
Contributor: ilia
Publication Year: 2024
DOI: https://doi.org/10.25950/888f9943
Computes the elastic constants for an arbitrary crystal. A robust computational protocol is used, attempting multiple methods and step sizes to achieve an acceptably low error in numerical differentiation and deviation from material symmetry. The crystal structure is specified using the AFLOW prototype designation as part of the Crystal Genome testing framework. In addition, the distance from the obtained elasticity tensor to the nearest isotropic tensor is computed.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Elastic constants for MgY in AFLOW crystal prototype A24B5_cI58_217_2g_ac at zero temperature and pressure v000 | view | 435771 | |
| Elastic constants for MgY in AFLOW crystal prototype A2B_hP12_194_ah_f at zero temperature and pressure v000 | view | 261633 |
Creators: Junhao Li and Ellad Tadmor
Contributor: tadmor
Publication Year: 2019
DOI: https://doi.org/10.25950/5853fb8f
Computes the cubic elastic constants for some common crystal types (fcc, bcc, sc, diamond) by calculating the hessian of the energy density with respect to strain. An estimate of the error associated with the numerical differentiation performed is reported.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Elastic constants for bcc Mg at zero temperature v006 | view | 27460 | |
| Elastic constants for bcc Y at zero temperature v006 | view | 24845 | |
| Elastic constants for diamond Mg at zero temperature v001 | view | 87903 | |
| Elastic constants for fcc Mg at zero temperature v006 | view | 38541 | |
| Elastic constants for fcc Y at zero temperature v006 | view | 23343 | |
| Elastic constants for sc Mg at zero temperature v006 | view | 47353 | |
| Elastic constants for sc Y at zero temperature v006 | view | 28396 |
Creators:
Contributor: ilia
Publication Year: 2024
DOI: https://doi.org/10.25950/2f2c4ad3
Computes the equilibrium crystal structure and energy for an arbitrary crystal at zero temperature and applied stress by performing symmetry-constrained relaxation. The crystal structure is specified using the AFLOW prototype designation. Multiple sets of free parameters corresponding to the crystal prototype may be specified as initial guesses for structure optimization. No guarantee is made regarding the stability of computed equilibria, nor that any are the ground state.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Equilibrium crystal structure and energy for MgY in AFLOW crystal prototype A2B_hP12_194_ah_f v002 | view | 96369 | |
| Equilibrium crystal structure and energy for MgY in AFLOW crystal prototype AB_cP2_221_a_b v002 | view | 67444 |
Creators:
Contributor: ilia
Publication Year: 2025
DOI: https://doi.org/10.25950/866c7cfa
Computes the equilibrium crystal structure and energy for an arbitrary crystal at zero temperature and applied stress by performing symmetry-constrained relaxation. The crystal structure is specified using the AFLOW prototype designation. Multiple sets of free parameters corresponding to the crystal prototype may be specified as initial guesses for structure optimization. No guarantee is made regarding the stability of computed equilibria, nor that any are the ground state.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Equilibrium crystal structure and energy for MgY in AFLOW crystal prototype A24B5_cI58_217_2g_ac v003 | view | 401760 | |
| Equilibrium crystal structure and energy for Mg in AFLOW crystal prototype A_cF4_225_a v003 | view | 152751 | |
| Equilibrium crystal structure and energy for Y in AFLOW crystal prototype A_cF4_225_a v003 | view | 155789 | |
| Equilibrium crystal structure and energy for Mg in AFLOW crystal prototype A_cI2_229_a v003 | view | 169749 | |
| Equilibrium crystal structure and energy for Mg in AFLOW crystal prototype A_hP2_194_c v003 | view | 177355 | |
| Equilibrium crystal structure and energy for Y in AFLOW crystal prototype A_hP2_194_c v003 | view | 187364 |
Creators: Daniel S. Karls and Junhao Li
Contributor: karls
Publication Year: 2019
DOI: https://doi.org/10.25950/2765e3bf
Equilibrium lattice constant and cohesive energy of a cubic lattice at zero temperature and pressure.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Equilibrium zero-temperature lattice constant for bcc Mg v007 | view | 12960 | |
| Equilibrium zero-temperature lattice constant for diamond Mg v007 | view | 13198 | |
| Equilibrium zero-temperature lattice constant for diamond Y v007 | view | 12989 | |
| Equilibrium zero-temperature lattice constant for fcc Mg v007 | view | 15534 | |
| Equilibrium zero-temperature lattice constant for fcc Y v007 | view | 13079 | |
| Equilibrium zero-temperature lattice constant for sc Mg v007 | view | 12751 | |
| Equilibrium zero-temperature lattice constant for sc Y v007 | view | 17374 |
Creators: Daniel S. Karls and Junhao Li
Contributor: karls
Publication Year: 2019
DOI: https://doi.org/10.25950/c339ca32
Calculates lattice constant of hexagonal bulk structures at zero temperature and pressure by using simplex minimization to minimize the potential energy.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Equilibrium lattice constants for hcp Mg v005 | view | 248740 | |
| Equilibrium lattice constants for hcp Y v005 | view | 244920 |
Creators:
Contributor: efuem
Publication Year: 2023
DOI: https://doi.org/10.25950/fca89cea
Computes the monovacancy formation energy and relaxation volume for cubic and hcp monoatomic crystals.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Monovacancy formation energy and relaxation volume for hcp Mg | view | 784353 | |
| Monovacancy formation energy and relaxation volume for hcp Y | view | 588228 |
Creators:
Contributor: efuem
Publication Year: 2023
DOI: https://doi.org/10.25950/c27ba3cd
Computes the monovacancy formation and migration energies for cubic and hcp monoatomic crystals.
| Test | Test Results | Link to Test Results page | Benchmark time
Usertime multiplied by the Whetstone Benchmark. This number can be used (approximately) to compare the performance of different models independently of the architecture on which the test was run.
Measured in Millions of Whetstone Instructions (MWI) |
|---|---|---|---|
| Vacancy formation and migration energy for hcp Mg | view | 973042 | |
| Vacancy formation and migration energy for hcp Y | view | 1420728 |
| Test | Error Categories | Link to Error page |
|---|---|---|
| Elastic constants for MgY in AFLOW crystal prototype A24B5_cI58_217_2g_ac at zero temperature and pressure v000 | other | view |
ElasticConstantsCubic__TD_011862047401_006
| Test | Error Categories | Link to Error page |
|---|---|---|
| Elastic constants for diamond Y at zero temperature v001 | other | view |
ElasticConstantsHexagonal__TD_612503193866_004
| Test | Error Categories | Link to Error page |
|---|---|---|
| Elastic constants for hcp Mg at zero temperature v004 | other | view |
| Elastic constants for hcp Y at zero temperature v004 | other | view |
EquilibriumCrystalStructure__TD_457028483760_003
| Test | Error Categories | Link to Error page |
|---|---|---|
| Equilibrium crystal structure and energy for Y in AFLOW crystal prototype A_aP1_2_a v003 | other | view |
| MEAM_LAMMPS_KimJeonLee_2015_MgY__MO_018428823000_002.txz | Tar+XZ | Linux and OS X archive |
| MEAM_LAMMPS_KimJeonLee_2015_MgY__MO_018428823000_002.zip | Zip | Windows archive |
This Model requires a Model Driver. Archives for the Model Driver MEAM_LAMMPS__MD_249792265679_002 appear below.
| MEAM_LAMMPS__MD_249792265679_002.txz | Tar+XZ | Linux and OS X archive |
| MEAM_LAMMPS__MD_249792265679_002.zip | Zip | Windows archive |